machine table must move to rotate the spindle of the
dividing head one revolution. Most milling machines
have a table screw of 4 threads per inch with a lead of
or
0.250 inch (1/4 inch) and a dividing head (index head)
with a 40:1 worm-to-spindle ratio. When the index head
is connected to the table through a 1:1 ratio, it will cut
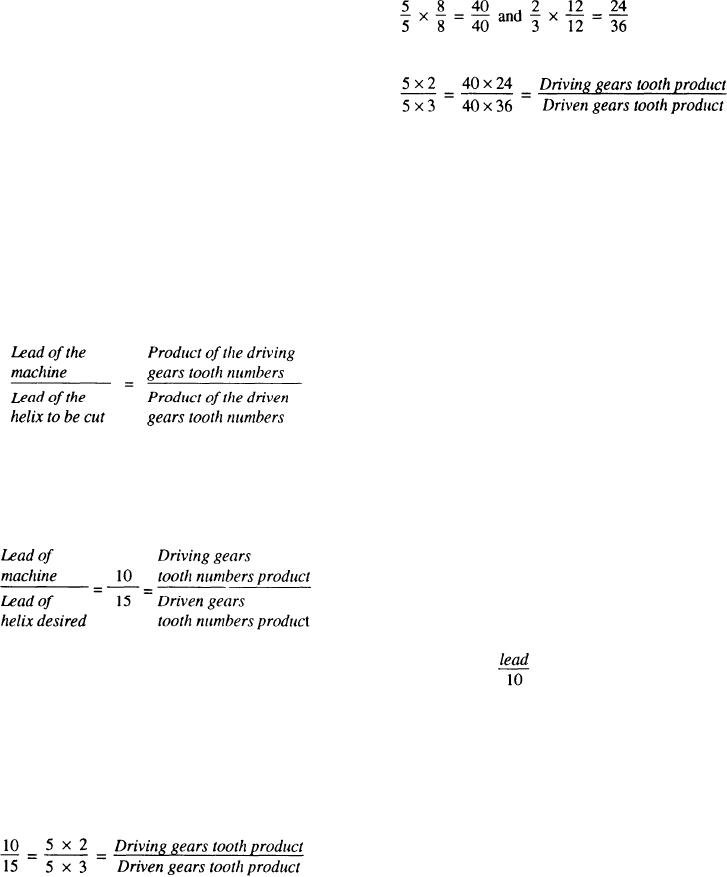
a lead of 10 inches. Thus, 40 turns of the lead screw are
Thus, gears with 40 and 24 teeth become the driving
required to make the spindle revolve one complete
gears, and gears with 40 and 36 teeth become the driven
revolution (40 0.250 inch = 10 inches). Therefore,
gears.
10 will be the constant in our gear train ratio formula,
These gears would be arranged in the gear train as
follows:
Gear A (on the dividing-head worm shaft)
All ratios other than 1:1 require modification of the gear
40 teeth (driven)
train.
Gear B (first gear on the idler stud)
From this formula, we can also say that the
24 teeth (driving)
Gear C (second gear on the idler stud)
36 teeth (driven)
Gear D (gear on the table screw)
40 teeth (driving)
Example:
The positions of the driving gears may be
Determine the change gears required for a lead of
interchanged without changing their products. The
15 inches. Assume the milling machine has a lead of 10
same is true of the driven gears. Thus, several different
inches.
a helix with the same lead.
Before you start to figure your change gear, check
your office library for a ready-made table for the
selection of gears devised by the Cincinnati Milling
Machine Company. These gears have been determined
If you could use a simple gear train (one driving and
using the formula,
. If you have already calculated
one driven gear), a lo-tooth gear on the table screw
meshed with a 15-tooth gear on the dividing-head worm
your lead, match it with the lead in the table and select
shaft would produce the 15-inch lead required.
the gears for that lead.
However, gears of 10 and 15 teeth are not available, and
the drive system is designed for a compound gear train
MANUFACTURING A HELICAL GEAR
of four gears. Therefore, the fraction 10/15 must be split
into two fractions whose product equals 10/15. Do this
by factoring as follows:
At this point of the chapter, you are ready to
manufacture a helical gear. In a case where you must
manufacture a helical gear from a sample, you should
do the following:
If gears with 5 and 2 teeth were possible, they would
1. Find the DP.
be the driving gears, and gears with 5 and 3 teeth would
2. Measure the OD. This is also the ROD.
be the driven gears. But since this is not possible, each
of the fractions must be expanded by multiplying both
3. Find the ADD.
the numerator and the denominator by a number that
4. Find the RPD.
will result in a product that corresponds to the number
of teeth on available gears:
5. Find the NT.
14-13