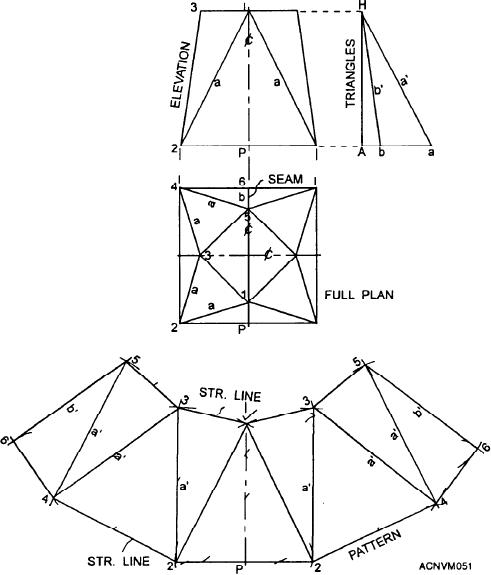
Figure 12-51.--Triangulation development of a twisted square.
8. Set your dividers for the distance between
4. Using the procedure for finding the length of
line b, measure the distance between point 4 and point
points 1 and 3 of the full plan. Using point 1 of the
5 to get the length of line a.
pattern for a pivot point, scribe an arc on each side of
point 1.
5. Draw an extension to the vertical center line of
the full-plan view. Construct a base line perpendicular
9. With a as the radius, and using each point 2 as
to the center line. Mark the intersection of the two lines
a pivot point in the pattern, scribe an arc to intersect each
in the pattern as point P
of two preceding arcs to establish points 3.
6. Use your dividers to measure the distance from
10. With 2-4 of the full plan as a radius, and
point P to point 2 in the full-plan view. Using this
using points 2 in the pattern for pivot points, scribe arcs
measurement as your radius and point P of your pattern
at 4.
as the pivot point, scribe an arc on each side of point P
11. Set the dividers for the length of a, and with
to get the true length of the base line. Label each of these
points 3 in the pattern for pivot points, scribe arcs to
points as point 2.
establish points 4.
7. Using the true length of line a of step 3 as your
12. Set the dividers for the distance between 3 and
radius, scribe an arc from each point 2 to intersect the
5 in the full plan. Using points 3 of the pattern for your
center line to establish point 1 and your first triangle in
pivot points, scribe arcs at 5.
the pattern.
12-21



